Physics 434, 2012: Lecture 16

Back to the main Teaching page.

Back to Physics 434, 2012: Information Processing in Biology. We are proceeding with the dynamical information processing block. In the next couple of lectures, we will follow the article by Detwiler et al., 2000.

Main lecture

1. We follow Detwiler et al., 2000, article in this lecture.
2. We discussed the basic structure of the vertebrate eye, which consists of rods and cones. We will return to the rods, and their unbelievable ability to detect single photons, in one of the later lectures.
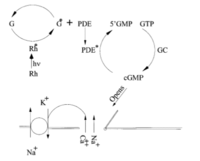
3. Crudely, signaling in vertebrate cones can be summarized by the following Fig. 2 of Detwiler et al., see figure on the right.
   

   Cones signaling
4. Notice the number of signaling elements known as futile cycles or push-pull enzymatic amplifiers in this system, see figure to the right.
   

   Enzymatic amplifier
   Why are these signaling elements so common in eukaryotic systems?
5. We start with the kinetic description of such enzymatic amplifier: ${\frac {dX^{*}}{dt}}=F-G={\frac {k_{a}E_{a}X}{1+X/K_{a}}}-{\frac {k_{d}E_{d}X^{*}}{1+X/K_{d}}}$. Notice that we use slightly different notation compared to the previous lecture.
6. Let's assume now that all enzymes are unsaturated, so that ${\frac {dX^{*}}{dt}}\approx k_{a}E_{a}X-k_{d}E_{d}X^{*}$.
7. We can now apply the steady state assumption and set: ${\frac {dX^{*}}{dt}}\approx k_{a}{\bar {E_{a}}}{\bar {X}}-k_{d}{\bar {E_{d}}}{\bar {X^{*}}}=0$. Here the overbar means the value averaged over long times. We further recall that $X^{*}+X=X_{\rm {tot}}$. This gives us ${\bar {X^{*}}}\approx {\frac {k_{a}{\bar {E_{a}}}X_{\rm {tot}}}{k_{a}{\bar {E_{a}}}+k_{d}{\bar {E_{d}}}}}.$
8. Recall that the input signal here is $E_{a}$ and the output is $X^{*}$. The form of the relation between the two indicated above is clearly the usual Michaelis-Menten curve.
9. Note that each activation-deactivation cycle consumes energy, and there are $k_{a}{\bar {E_{a}}}{\bar {X}}$ such cycles per unit time. Thus even at the steady state the system is not stationary and keeps on recirculating $X$, consuming energy. Why would this structure then be useful instead of a simpler arrangement, where $X$ is produced on demand? Activating a product is typically much faster than producing it, and hence the futile cycle can respond to a changing stimulus much quicker than the time that would be needed to transcribe and then translate the protein. Another reason is that this futile cycle structure is very tunable, as we will see below.
10. Now suppose $E_{a}={\bar {E}}_{a}+\Delta E_{a}$ and $X^{*}={\bar {X}}^{*}+\Delta X^{*}$. For simplicity, we assume that $E_{d}={\rm {const}}$. We can now expand our equations to the first order in fluctuations of the signal and the response.
    • ${\frac {d{\bar {X}}^{*}}{dt}}+{\frac {d\Delta X^{*}}{dt}}=F({\bar {X}}^{*},{\bar {E}}_{a})-G({\bar {X}}^{*},{\bar {E}}_{a})+{\frac {\partial F({\bar {X}}^{*},{\bar {E}}_{a})}{\partial X^{*}}}\Delta X^{*}+{\frac {\partial F({\bar {X}}^{*},{\bar {E}}_{a})}{\partial E_{a}}}\Delta E_{a}+{\frac {\partial G({\bar {X}}^{*},{\bar {E}}_{a})}{\partial X^{*}}}\Delta X^{*}+{\frac {\partial G({\bar {X}}^{*},{\bar {E}}_{a})}{\partial E_{a}}}\Delta E_{a}$
    • This results in ${\frac {d\Delta X^{*}}{dt}}={\frac {1}{\tau }}\left(\Delta X^{*}-g_{0}\Delta E_{a}\right)$, where $\tau =\left(k_{a}{\bar {E}}_{a}+k_{d}{\bar {E}}_{d}\right)^{-1}$, and $g_{0}=\tau k_{a}{\bar {X}}$.
11. From the differential equation description, it is clear that the system acts as a filter: without the signal, the response relaxes to $\Delta X^{*}=0$, and a change in the signal causes a delayed response in the output, with a delay of order of $\tau$. Thus the system smoothes out (filters) the signals to produce the response. Because $\tau$ defines the delay, $1/\tau$ is called the bandwidth" -- signals that change on scales faster than that simply "do not go through" the system.
12. Notice that if $\Delta E_{a}={\rm {const}}$, then by setting $d/dt=0$, we calculate the steady state of the system $\Delta X^{*}=g_{0}\Delta E_{a}$. Thus $g_{0}$ is called the static gain -- a response of the circuit at the steady state is $g_{0}$ times the signal.